ALAFF Modified Gram-Schmidt (MGS)

Subsection 3.2.4 Modified Gram-Schmidt (MGS)

In the video, we reasoned that the following two algorithms compute the same values, except that the columns of $Q $ overwrite the corresponding columns of $A \text{:}$

$\begin{array}{l} {\bf for~} j=0, \ldots, n-1 \\ ~~~ a_j^\perp := a_j \\ ~~~ {\bf for~} k=0, \dots, j-1 \\ ~~~ ~~~ \rho_{k,j} := q_k^H a_j^\perp \\ ~~~ ~~~ a_j^\perp := a_j^\perp - \rho_{k,j} q_k \\ ~~~ {\bf end} \\ ~~~ \rho_{j,j} := \| {a}_j^\perp \|_2 \\ ~~~ q_j := a_j^\perp / \rho_{j,j} \\ {\bf end} \end{array}$

$~$

$\begin{array}{l} {\bf for~} j=0, \ldots, n-1 \\ ~~\\ ~~~ {\bf for~} k=0, \dots, j-1 \\ ~~~ ~~~ \rho_{k,j} := a_k^H a_j \\ ~~~ ~~~ a_j := a_j - \rho_{k,j} a_k \\ ~~~ {\bf end} \\ ~~~ \rho_{j,j} := \| {a}_j \|_2 \\ ~~~ a_j := a_j / \rho_{j,j} \\ {\bf end} \end{array}$

(a) MGS algorithm that computes $Q $ and $R $ from $A \text{.}$

$~$

(b) MGS algorithm that computes $Q $ and $R $ from $A \text{,}$ overwriting $A $ with $Q\text{.}$

Homework 3.2.4.1.

Assume that $q_0, \ldots, q_{k-1} $ are mutually orthonormal. Let $\rho_{j,k} = q_j^H y $ for $j = 0, \ldots, i-1 \text{.}$ Show that

\begin{equation*} \begin{array}[t]{c} \underbrace{ q_i^H y } \\ \rho_{i,k} \end{array} = q_i^H \left( y - \rho_{0,k} q_0 - \cdots - \rho_{i-1,k} q_{i-1} \right) \end{equation*}

for $i = 0, \ldots, k-1 \text{.}$

Solution

\begin{equation*} \begin{array}{l} q_i^H \left( y - \rho_{0,k} q_0 - \cdots - \rho_{i-1,k} q_{i-1} \right) \\ ~~~=~~~~ \lt \mbox{ distribute } \gt \\ q_i^H y - q_i^H \rho_{0,k} q_0 - \cdots - q_i^H \rho_{i-1,k} q_{i-1} \\ ~~~=~~~~ \lt \rho_{0,k} \mbox{ is a scalar } \gt \\ q_i^H y - \rho_{0,k} \begin{array}[t]{c} \underbrace{ q_i^H q_0} \\ 0 \end{array} - \cdots - \begin{array}[t]{c} \underbrace{ \rho_{i-1,k} q_i^H q_{i-1} } \\ 0 \end{array} \end{array} \end{equation*}

This homework illustrates how, given a vector $y \in \Cm $ and a matrix $Q \in \C^{mxk} $ the component orthogonal to the column space of $Q \text{,}$ given by $( I - Q Q^H ) y \text{,}$ can be computed by either of the two algorithms given in Figure 3.2.4.1. The one on the left, $\mbox{Proj}\perp Q_{\rm CGS}( Q, y ) $ projects $y$ onto the column space perpendicular to $Q $ as did the Gram-Schmidt algorithm with which we started. The one on the left successfuly subtracts out the component in the direction of $q_i $ using a vector that has been updated in previous iterations (and hence is already orthogonal to $q_0, \ldots, q_{i-1} $). The algorithm on the right is one variant of the Modified Gram-Schmidt (MGS) algorithm.

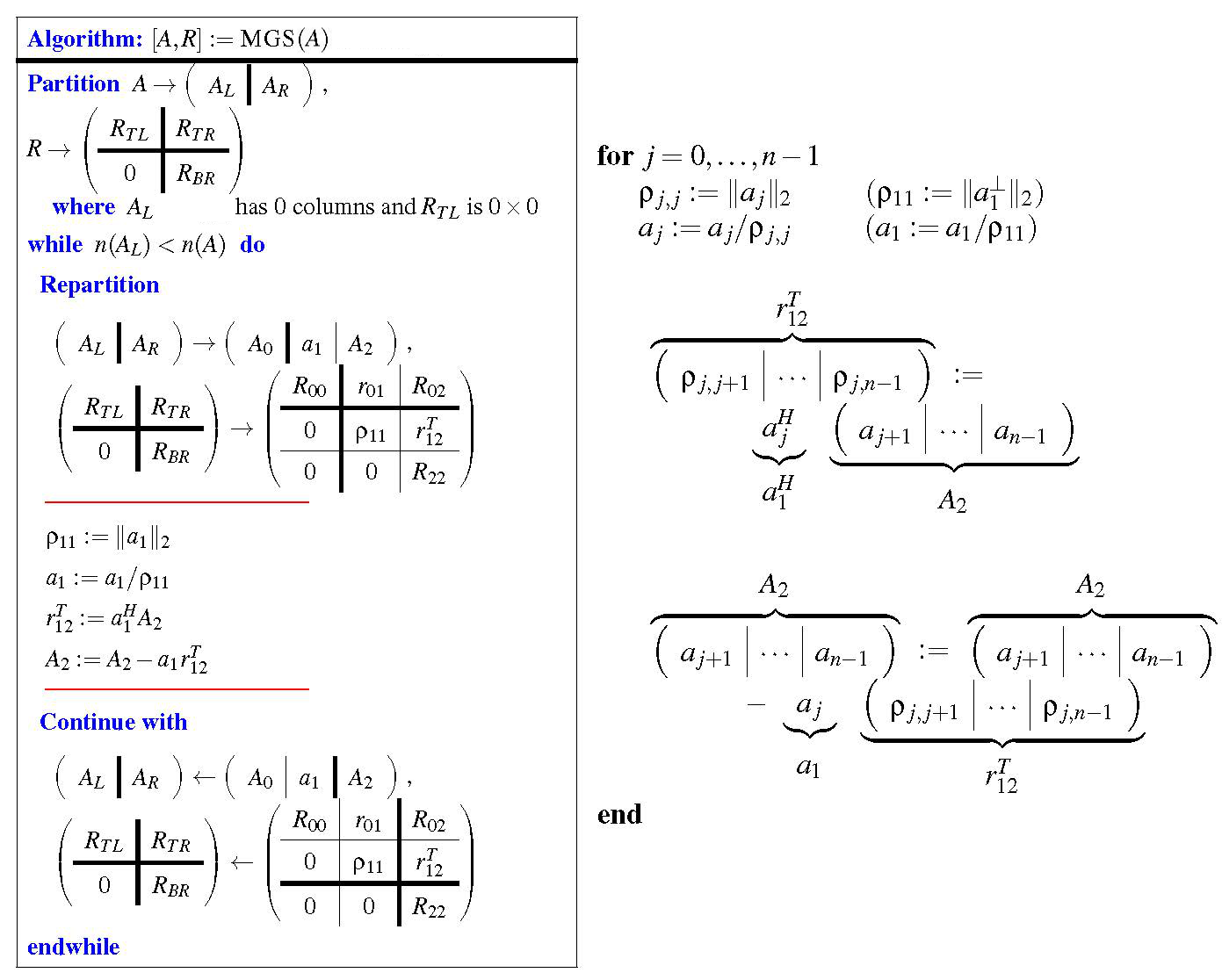
\begin{equation*} \begin{array}{|l | l|} \hline [ y^\perp, r ] = {\rm Proj\!\!\perp\!\! Q}_{\rm CGS}( Q, y ) ~~~~~~~~~~~~ \amp [ y^\perp, r ] = {\rm Proj\!\!\perp\!\! Q}_{\rm MGS}( Q, y ) ~~~~~~~~~~~~ \\ {\rm (used~by~CGS)} \amp {\rm (used~by~MGS)} \\ \hline y^\perp = y \amp y^\perp = y \\ {\bf for~} i=0, \ldots, k-1 \amp {\bf for~} i=0, \ldots, k-1 \\ ~~~\rho_i := q_i^H y \amp ~~~ \rho_i := q_i^H y^\perp \\ ~~~ y^\perp := y^\perp - \rho_i q_i \amp ~~~ y^\perp := y^\perp - \rho_i q_i \\ {\bf endfor} \amp {\bf endfor} \\ \hline \end{array} \end{equation*}

Figure 3.2.4.1. Two different ways of computing $y^\perp = ( I - Q Q^H ) y = y - Q r \text{,}$ where $r = Q^H y \text{.}$ The computed $y^\perp $ is the component of $y $ orthogonal to ${\cal C}(Q) \text{,}$ where $Q $ has $k $ orthonormal columns. (Notice the $y $ on the left versus the $y^\perp $ on the right in the computation of $\rho_i \text{.}$)

These insights allow us to present CGS and this variant of MGS in FLAME notation, in Figure 3.2.4.2 (left and middle).

\begin{equation*} \newcommand{\operation}{ \left[Q, R \right] := \mbox{Gram-Schmidt}( A ) } \newcommand{\routinename}{ \left[ A, R \right] := \mbox{GS}( A ) \quad \mbox{ (overwrites $ A $ with $ Q $)}} % Step 3: Loop-guard \newcommand{\guard}{ n( A_L ) \lt n( A ) } % Step 4: Initialize \newcommand{\partitionings}{ A \rightarrow \FlaOneByTwo{A_L}{A_R} , R \rightarrow \FlaTwoByTwo{R_{TL}}{R_{TR}} {0}{R_{BR}} } \newcommand{\partitionsizes}{ A_L {\rm ~has~} 0 {\rm ~columns~and~} R_{TL} {\rm ~is~} 0 \times 0 } % Step 5a: Repartition the operands \newcommand{\repartitionings}{ \FlaOneByTwo{A_L}{A_R} \rightarrow \FlaOneByThreeR{A_0}{a_1}{A_2} , \FlaTwoByTwo{R_{TL}}{R_{TR}} {0}{R_{BR}} \rightarrow \FlaThreeByThreeBR{R_{00}}{r_{01}}{R_{02}} {0}{\rho_{11}}{r_{12}^T} {0}{0}{R_{22}} } % \newcommand{\repartitionsizes}{ % a_1 {\rm ~and~} q_1 {\rm ~are~columns,~} % \rho_{11} {\rm is~a~scalar} % } % Step 5b: Move the double lines \newcommand{\moveboundaries}{ \FlaOneByTwo{A_L}{A_R} \leftarrow \FlaOneByThreeL{A_0}{a_1}{A_2} , \FlaTwoByTwo{R_{TL}}{R_{TR}} {0}{R_{BR}} \leftarrow \FlaThreeByThreeTL{R_{00}}{r_{01}}{R_{02}} {0}{\rho_{11}}{r_{12}^T} {0}{0}{R_{22}} } % Step 8: Insert the updates required to change the % state from that given in Step 6 to that given in Step 7 % Note: The below needs editing!!! \newcommand{\update}{ \begin{array}{l | l | l} \hline {\rm CGS} \amp {\rm MGS} \amp {\rm MGS~(alternative)} \\ r_{01} := A_0^H a_1 \amp \amp \\ a_1 := a_1 - A_0 r_{01} \amp [ a_1, r_{01} ] = {\rm Proj\!\!\perp\!\! toQ}_{\rm MGS}( A_0, a_1 ) \amp \\ \rho_{11} := \| a_1 \|_2 \amp \rho_{11} := \| a_1 \|_2 \amp \rho_{11} := \| a_1 \|_2 \\ a_1 := a_1 / \rho_{11} \amp a_1 := a_1 / \rho_{11} \amp a_1 := a_1 / \rho_{11} \\ \amp \amp r_{12}^T := a_1^H A_2 \\ \amp \amp A_2 := A_2 - a_1 r_{12}^T \\ \hline \end{array} } \FlaAlgorithm % this command generates the worksheet using the % commands that were defined between the \resetsteps % and the worksheet command \end{equation*}

Figure 3.2.4.2. Left: Classical Gram-Schmidt algorithm. Middle: Modified Gram-Schmidt algorithm. Right: Alternative Modified Gram-Schmidt algorithm. In this last algorithm, every time a new column, $q_1 \text{,}$ of $Q $ is computed, each column of $A_2 $ is updated so that its component in the direction of $q_1 $ is is subtracted out. This means that at the start and finish of the current iteration, the columns of $A_L $ are mutually orthonormal and the columns of $A_R $ are orthogonal to the columns of $A_L \text{.}$

Next, we massage the MGS algorithm into the alternative MGS algorithmic variant given in Figure 3.2.4.2 (right).

The video discusses how MGS can be rearranged so that every time a new vector $q_k $ is computed (overwriting $a_k $), the remaining vectors, $\{ a_{k+1}, \ldots, a_{n-1} \} \text{,}$ can be updated by subtracting out the component in the direction of $q_k \text{.}$ This is also illustrated through the next sequence of equivalent algorithms.

$\begin{array}{l} {\bf for~} j=0, \ldots, n-1 \\ ~~~ \rho_{j,j} := \| {a}_j \|_2 \\ ~~~ a_j := a_j / \rho_{j,j} \\ ~~~ {\bf for~} k=j+1, \dots, n-1 \\ ~~~ ~~~ \rho_{j,k} := a_j^H a_k \\ ~~~ ~~~ a_k := a_k - \rho_{j,k} a_j \\ ~~~ {\bf end} \\ {\bf end} \end{array}$

$~$

$\begin{array}{l} {\bf for~} j=0, \ldots, n-1 \\ ~~~ \rho_{j,j} := \| {a}_j \|_2 \\ ~~~ a_j := a_j / \rho_{j,j} \\ ~~~ {\bf for~} k=j+1, \dots, n-1 \\ ~~~ ~~~ \rho_{j,k} := a_j^H a_k \\ ~~~ {\bf end} \\ ~~~ {\bf for~} k=j+1, \dots, n-1 \\ ~~~ ~~~ a_k := a_k - \rho_{j,k} a_j \\ ~~~ {\bf end} \\ {\bf end} \end{array}$

(c) MGS algorithm that normalizes the $j $th column to have unit length to compute $q_j $ (overwriting $a_j $ with the result) and then subtracts the component in the direction of $q_j $ off the rest of the columns $(a_{j+1}, \ldots , a_{n-1})\text{.}$

$~$

(d) Slight modification of the algorithm in (c) that computes $\rho_{j,k} $ in a separate loop.

$\begin{array}{l} {\bf for~} j=0, \ldots, n-1 \\ ~~~ \rho_{j,j} := \| {a}_j \|_2 \\ ~~~ a_j := a_j / \rho_{j,j} \\ ~~~ ~~~ \left( \begin{array}{c | c | c} \rho_{j,j+1} \amp \cdots \amp \rho_{j,n-1} \end{array} \right) := \\ ~~~ ~~~ a_j^H \left( \begin{array}{c | c | c} a_{j+1} \amp \cdots \amp a_{n-1} \end{array} \right) \\ ~~~ \left( \begin{array}{c | c | c} a_{j+1} \amp \cdots \amp a_{n-1} \end{array} \right) := \\ ~~~ ~~~ \left( \begin{array}{c | c | c} a_{j+1} - \rho_{j,j+1} a_j \amp \cdots \amp a_{n-1} - \rho_{j,n-1} a_j \end{array} \right) \\ {\bf end} \end{array}$

$~$

$\begin{array}{l} {\bf for~} j=0, \ldots, n-1 \\ ~~~ \rho_{j,j} := \| {a}_j \|_2 \\ ~~~ a_j := a_j / \rho_{j,j} \\ ~~~ \left( \begin{array}{c | c | c} \rho_{j,j+1} \amp \cdots \amp \rho_{j,n-1} \end{array} \right) := \\ ~~~ ~~~ a_j^H \left( \begin{array}{c | c | c} a_{j+1} \amp \cdots \amp a_{n-1} \end{array} \right) \\ ~~~ \left( \begin{array}{c | c | c} a_{j+1} \amp \cdots \amp a_{n-1} \end{array} \right):= \\ ~~~ ~~~ \left( \begin{array}{c | c | c} a_{j+1} \amp \cdots \amp a_{n-1} \end{array} \right) \\ ~~~ ~~~ ~~~ - a_j \left( \begin{array}{c | c | c} \rho_{j,j+1} \amp \cdots \amp \rho_{j,n-1} \end{array} \right) \\ {\bf end} \end{array}$

(e) Algorithm in (d) rewritten to expose only the outer loop.

$~$

(f) Algorithm in (e) rewritten to expose the row-vector-times matrix multiplication $a_j^H \left( \begin{array}{c | c | c } a_{j+1} \amp \cdots \amp a_{n-1} \end{array} \right) $ and rank-1 update $\left( \begin{array}{c | c | c } a_{j+1} \amp \cdots \amp a_{n-1} \end{array} \right) - a_j \left( \begin{array}{c | c | c } \rho_{j,j+1} \amp \cdots \amp \rho_{j,n-1} \end{array} \right) \text{.}$

Figure 3.2.4.3. Various equivalent MGS algorithms.

This discussion shows that the updating of future columns by subtracting out the component in the direction of the latest column of $Q $ to be computed can be cast in terms of a rank-1 update. This is also captured, using FLAME notation, in the algorithm in Figure 3.2.4.2, as is further illustrated in Figure 3.2.4.4:

Figure 3.2.4.4. Alternative Modified Gram-Schmidt algorithm for computing the QR factorization of a matrix $A \text{.}$

Ponder This 3.2.4.2.

Let $A $ have linearly independent columns and let $A = Q R $ be a QR factorization of $A \text{.}$ Partition

\begin{equation*} A \rightarrow \FlaOneByTwo{A_L}{A_R}, \quad Q \rightarrow \FlaOneByTwo{Q_L}{Q_R}, {\rm ~~~and~~~} R \rightarrow \FlaTwoByTwo {R_{TL}}{R_{TR}} { 0 }{R_{BR}}, \end{equation*}

where $A_L $ and $Q_L $ have $k $ columns and $R_{TL} $ is $k \times k \text{.}$

As you prove the following insights, relate each to the algorithm in Figure 3.2.4.4. In particular, at the top of the loop of a typical iteration, how have the different parts of $A $ and $R $ been updated?

$A_L = Q_L R_{TL}\text{.}$

($Q_L R_{TL} $ equals the QR factorization of $A_L \text{.}$)
${\cal C}( A_L ) = {\cal C}( Q_L ) \text{.}$

(The first $k$ columns of $Q $ form an orthonormal basis for the space spanned by the first $k $ columns of $A \text{.}$)
$R_{TR} = Q_L^H A_R\text{.}$
$(A_R - Q_L R_{TR} )^H Q_L = 0 \text{.}$

(Each column in $A_R - Q_L R_{TR}$ equals the component of the corresponding column of $A_R $ that is orthogonal to $\Span( Q_L ) \text{.}$)
${\cal C}( A_R - Q_L R_{TR} ) = {\cal C}( Q_R ) \text{.}$
$A_R - Q_L R_{TR} = Q_R R_{BR} \text{.}$

(The columns of $Q_R $ form an orthonormal basis for the column space of $A_R - Q_L R_{TR} \text{.}$)

Solution

Consider the fact that $A = Q R \text{.}$ Then, multiplying the partitioned matrices,

\begin{equation*} \begin{array}{rcl} \FlaOneByTwo{A_L}{A_R} \amp = \amp \FlaOneByTwo{Q_L}{Q_R} \FlaTwoByTwo {R_{TL}}{R_{TR}} { 0 }{R_{BR}} \\ \amp = \amp \FlaOneByTwo{Q_L R_{TL}}{Q_L R_{TR} + Q_R R_{BR}}. \end{array} \end{equation*}

Hence

\begin{equation} A_L = Q_L R_{TL} \quad \mbox{and} \quad A_R = Q_L R_{TR} + Q_R R_{BR}.\label{MGS-eqn-1}\tag{3.2.2} \end{equation}

The left equality in (3.2.2) answers 1.
${\cal C}( A_L ) = {\cal C}( Q_L ) $ can be shown by noting that $R $ is upper triangular and nonsingular and hence $R_{TL} $ is upper triangular and nonsingular, and using this to show that ${\cal C}( A_L ) \subset {\cal C}( Q_L ) $ and ${\cal C}( Q_L ) \subset {\cal C}( A_L ) \text{:}$
- ${\cal C}( A_L ) \subset {\cal C}( Q_L ) \text{:}$ Let $y \in {\cal C}( A_L ) \text{.}$ Then there exists $x $ such that $A_L x = y \text{.}$ But then $Q_L R_{TL} x = y $ and hence $Q_L( R_{TL} x ) = y $ which means that $y \in {\cal C}( Q_L ) \text{.}$
- ${\cal C}( Q_L ) \subset {\cal C}( A_L ) \text{:}$ Let $y \in {\cal C}( Q_L ) \text{.}$ Then there exists $x $ such that $Q_L x = y \text{.}$ But then $A_L R_{TL}^{-1} x = y $ and hence $A_L( R_{TL}^{-1} x ) = y $ which means that $y \in {\cal C}( A_L ) \text{.}$
This answers 2.
Take $A_R - Q_L R_{TR} = Q_R R_{BR} $ and multiply both side by $Q_L^H \text{:}$

\begin{equation*} Q_L^H( A_R - Q_L R_{TR} )= Q_L^H Q_R R_{BR} \end{equation*}

is equivalent to

\begin{equation*} Q_L^H A_R - \begin{array}[t]{c} \underbrace{ Q_L^H Q_L } \\ I \end{array} R_{TR} = \begin{array}[t]{c} \underbrace{ Q_L^H Q_R } \\ 0 \end{array} R_{BR} = 0. \end{equation*}

Rearranging yields 3.
Since $A_R - Q_L R_{TR} = Q_R R_{BR} $ we find that $( A_R - Q_L R_{TR} )^H Q_L = ( Q_R R_{BR} )^H Q_L $ and

\begin{equation*} ( A_R - Q_L R_{TR} )^H Q_L = R_{BR}^H Q_R^H Q_L = 0. \end{equation*}
Similar to the proof of 2.
Rearranging the right equality in (3.2.2) yields $A_R - Q_L R_{TR} = Q_R R_{BR}. \text{,}$ which answers 5.
Letting $\widehat A $ denote the original contents of $A \text{,}$ at a typical point,
- $A_L $ has been updated with $Q_L \text{.}$
- $R_{TL} $ and $R_{TR} $ have been computed.
- $A_R = \widehat A_R - Q_L R_{TR} \text{.}$

Homework 3.2.4.3.

Implement the algorithm in Figure 3.2.4.4 as

function [ Aout, Rout ] = MGS_QR( A, R )

Input is an $m \times n $ matrix A and a $n \times n $ matrix $R \text{.}$ Output is the matrix $Q \text{,}$ which has overwritten matrix A, and the upper triangular matrix $R \text{.}$ (The values below the diagonal can be arbitrary.) You may want to use Assignments/Week03/matlab/test_MGS_QR.m to check your implementation.

Solution

See Assignments/Week03/answers/MGS_QR.m.